X-Treme Audio MISI - Manual (Page 4)

User’s manual
4.1 Infinite length linear source
With reference to fig. 1, let’s imagine an infinite sequence of unrelated
sources, spaced out by a distance b and all having the same sound
power Wo. As a result, the intensity of each source adds up to that of
the other sources in determining the total intensity at the distance ro in
a normal direction towards the linear source. Working from this hypoth-
esis, we can demonstrate (Beranek, 1988) that with a distance value
r
o
>= b/ π
and therefore at a distance such that the individual sources cannot
be distinguished one from the other, the
average sound intensity
can be simply approximated by the relationship:
〈I〉=Wo/4br
o
[W/m 2 ].
In other words, at distances exceeding the distance between one
source and the next (these are exactly the listening conditions of the
vertical line array sound reinforcement systems, since the dis-
tance between the elements is generally one linear metre) the inten-
sity no longer varies with the square of the distance as in the case of
the monopole sources, but is inversely proportional to the distance.
This means that these sound waves (often known as
cylindrical)
decrease by just 3 dB for each doubling of the distance rather than
by 6 dB as it occurs in traditional systems (the previously mentioned
“inverse-square” law).
4.2 Finite length linear source
If the sources arranged on a line are in a finite number n (>=3) and
βn is the angle, in radians, below which the source line is seen from
the observation point (as indicated in fig. 1), always at sufficiently
large distances (r
o
>= b/ π), the sound intensity can be determined
according to the following relationship:
〈I〉 = Wo·βn /4πbr
o
[W/m 2 ].
In this case too it is inversely proportional to the distance and,
obviously, it is directly proportional to the angle opening below
which the linear source is seen from the listening point. In conclu-
sion, in this case, one can state that a reasonably accurate de-
scription of the
vertical line array behaviour has been reached,
which is approximated as a finite linear source in its near field.
In fact, owing to the di mensions of the magnitudes at issue, the
b/π limit beyond which the array can be approximated as a linear
source (and therefore it can be seen as a source of cylindrical
waves) is much smaller than the above-mentioned limit between
a far field and a near field (it should be recalled that this limit is
represented by the larger distance between the following ones:
r >>
λ
max
/2 π, r >> L , r >> πL 2 /2 λ
max
).
Note: some empirical formulas can be found (Smith, Heil and
others) in which the border distance from the near to the far
field depends on variables such as the array length or the
reproduced frequency. However, it is better not to use them
as they lack any general validity!
In the far field, on the contrary, it is right to apply the considerations
mentioned just few lines earlier about the “inverse-square” law. In
particular, in the case of linear sources, since the sound power of a
single source Wo is known, the formula used to calculate the sound
pressure level in the free-field conditions on a reflecting surface will
be:
Lp = Lw
o
+ 10 log( βn /r) – 8 [dB],
where βn is the angle below which the sources are seen from the
listening point.
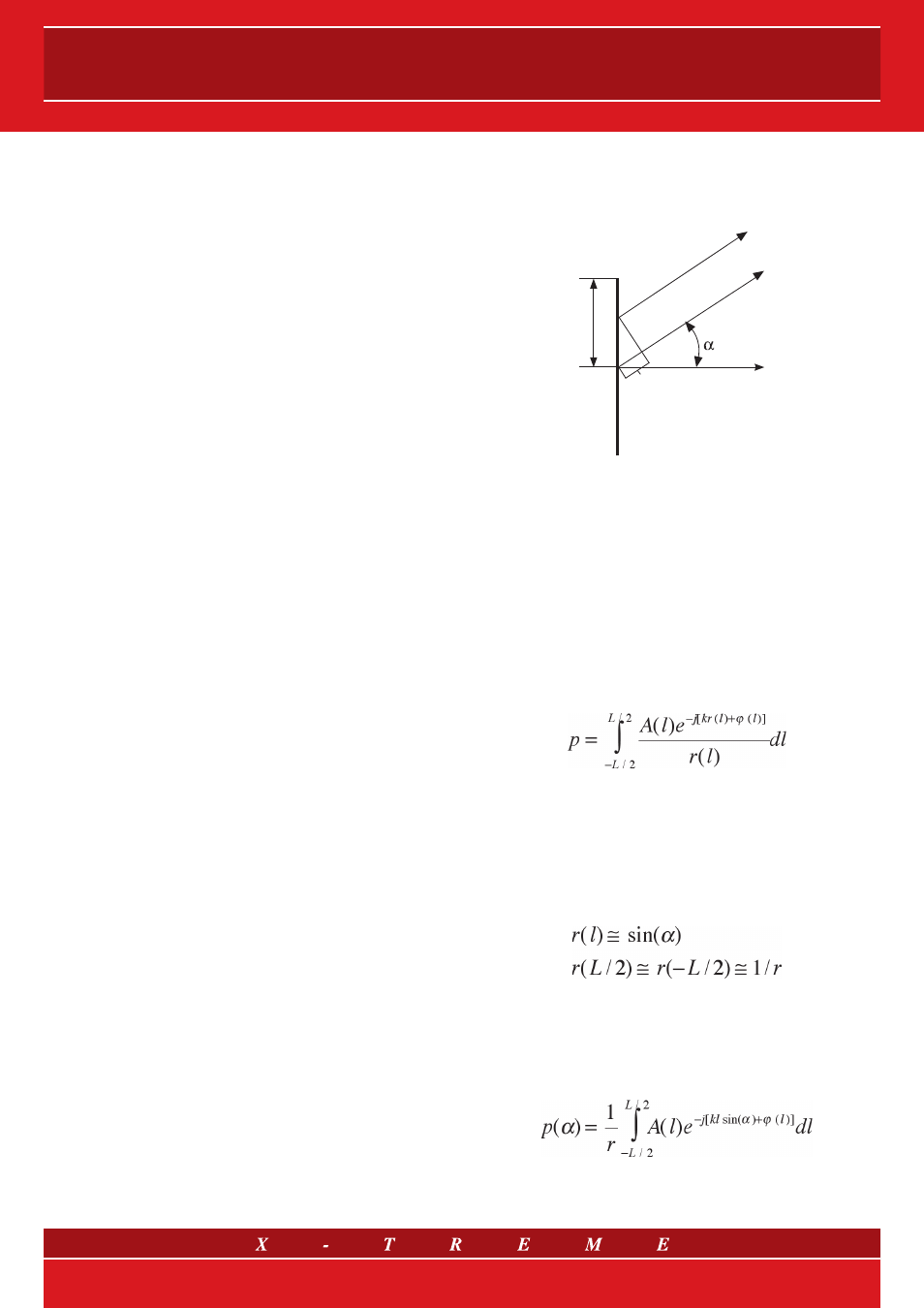
5. Description of the sound field of a linear source
In order to analyse the sound field generated by a line array let’s
start from a simple and ideal model: the
finite linear source (or
line source).
dl
dl sin( α)
x
Line source
Far field
L/2
fig. 2
The sound pressure generated by a linear source can be ob-
tained analytically as a special solution of the
wave equation,
in relation to space and time coordinates. Moreover, it is also
assumed that the source can only emit a sinusoidal signal. From
a mathematical viewpoint, this simplification allows us to use a
notation (known as
phasorial) which simplifies calculations and
ensures completeness without losing its general character. The
Fourier theory shows that, within some hypotheses (which have
been widely verified in the case of musical signals), any periodical
signal can be modelled as the sum of individual sinusoids.
Having said this, we can therefore express the sound pressure gen-
erated from a linear source as:
where L is the line length, k is the wave number, A(l) and ϕ (l) are
the signal amplitude and phase respectively on a point of the line (or
rather on an infinitesimal segment dl) at a distance r(l) from a generic
observation point or, rather, from a listening point P.
In order to analytically verify the line array properties, a few ad-
ditional hypotheses are required. For example, it can be easily no-
ticed that, beyond a certain observation (or ‘listening’) distance,
one will have:
This further condition is precisely that of the far field, as previously
mentioned.
Thanks to the far field hypothesis we can rewrite the final expression
of pressure in a form that we will use to evaluate the source directiv-
ity (see paragraph 5.1):
4/21